I am looking for a 4-bit S-box which has only 4s in its difference distribution table (except the top left corner) but I was not able to find one in the literature.
Has such an S-box been already published? If not, is it theoretically possible to reach this property? If so, how to proceed?
Yes. All $4\times4$ bit S-boxes have been classified. It is possible to reach a maximum differential of 4 as well as optimum nonlinearity.
SERPENT used optimal $4\times 4$ S-boxes with respect to differential cryptanalysis. So does PRESENT, to the best of my knowledge.
Some links
2011 - Markku-Juhani O. Saarinen, Cryptographic Analysis of All 4 × 4-Bit S-Boxes
2015 - Zhang et.al., A New Classification of 4-bit Optimal S-boxes and its Application to PRESENT, RECTANGLE and SPONGENT
SAGE is a good way to learn about and experiment with S-boxes.
Edit: None of the optimal Sboxes' representatives from Saarinen have the property of all 4's you want. I have no time, but I believe it can be proved using Theorem 1
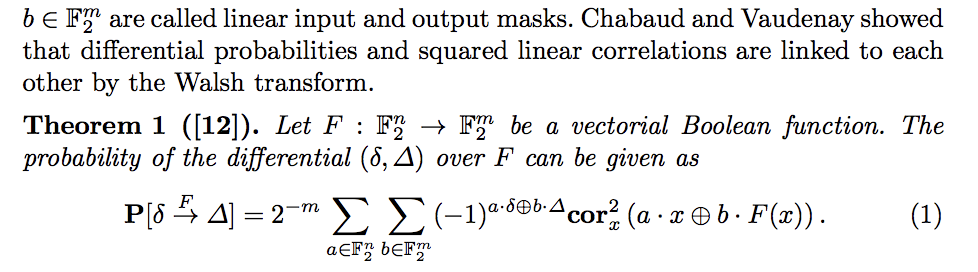
in Blondeau-Nyberg which originally appeared in Eurocrypt 94 Chabaud-Vaudenay that such a DDT pattern would yield weak S-boxes against linear cryptanalysis. Here $n=m=4,$ $cor_x(a\cdot x \oplus b\cdot F(x))$ is the correlation bias of the corresponding linear characteristic, and $P[\delta \stackrel{F}{\rightarrow} \Delta]$ is the diferential probability.
