1
$\begingroup$

Is AES GCM without GMAC vulnerable against bit-flip attacks? Let's assume the plaintext is known for some reason (e.g. it can be guessed). In my opinion, I can flip bits in the ciphered blocks and can so generate a plaintext, which is flipped at the same position. So it would be easy to change 0x01 to 0x00 in the plaintext, even when I don't know the key or Initialization vector.

Did I oversee something? enter image description here

Improve this question
$\endgroup$
8
  • 1
    $\begingroup$ Do you mean "Lets assume the plaintext is known"? $\endgroup$
    – Martin Thompson
    Commented Feb 7, 2022 at 14:15
  • 1
    $\begingroup$ HMAC ensures integrity, without it there is no way for the receiver to check that the ciphertext wasn't tampered with. Therefore, a number of attacks apply and in particular simple bitflips. $\endgroup$
    – Marc Ilunga
    Commented Feb 7, 2022 at 14:19
  • 3
    $\begingroup$ As far as I understand, GCM mode is defined to include the authentication code - from the spec "The two functions that comprise GCM are called authenticated encryption and authenticated decryption"... so without the GMAC, it is potentially vulnerable to all kinds of attacks. But you're not supposed to do that :) $\endgroup$
    – Martin Thompson
    Commented Feb 7, 2022 at 14:27
  • 1
    $\begingroup$ Seems my autocorrect prefers HMAC to GMAC. Anyway, the statement should still hold. An addition could be that without GMAC, we fallback on CTR. $\endgroup$
    – Marc Ilunga
    Commented Feb 7, 2022 at 14:34
  • 1
    $\begingroup$ If they are discussing 'lets do GCM without passing or validating the tag', they're effectively saying 'lets use a broken version of GCM'. If they don't/can't spend the bandwidth to send the GCM tag, they shouldn't be using GCM at all. $\endgroup$
    – poncho
    Commented Feb 7, 2022 at 15:47

1 Answer 1

Reset to default
2
$\begingroup$

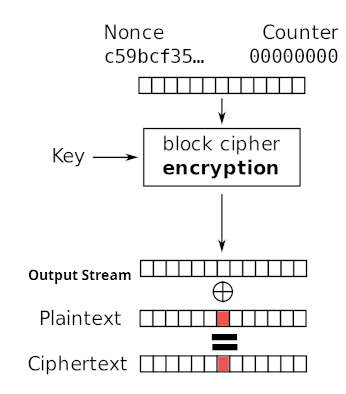
As an Authenticated Encryption with Associated Data, AES-GCM internally uses CTR mode and GCM adds authentication and integrity. Without GCM one can have only Ind-CPA security.

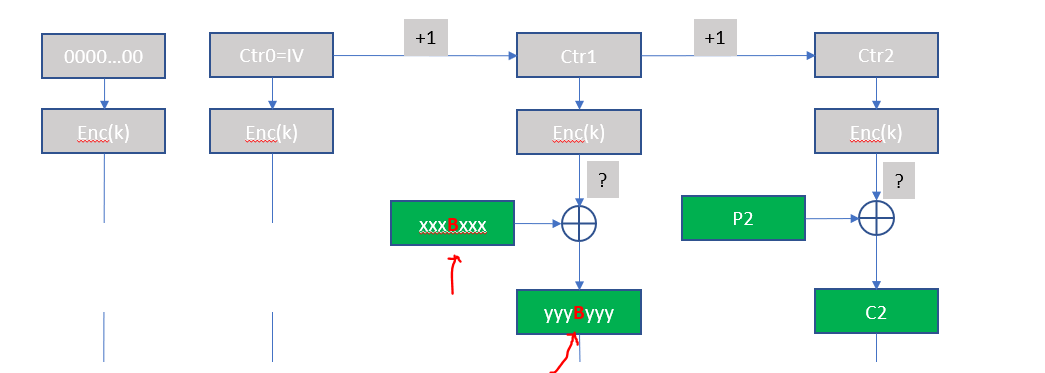
CTR in AES-GCM starts IV incremented ($inc_{32}(J_0)$ in NIST format and if IV size is not 96-bit then the IV is processed). The initial $J_0$ is used in the authentication tag calculation.

The rest is the usual CTR mode. If one knows the message, they can change the message into whatever message they want. Just x-or (the bit-flip attack) the necessary bits.

enter image description here

Consider the encryption. One has no control of the output stream. On the other hand, if the ciphertext is modified, only the plaintext is changed. Or see in the equation;

$$C_i = O_i \oplus P_i$$ The output stream $O_i$ is fixed by the block cipher encryption therefore changing the $C_i$ modifies the $P_i$.

This is, however, is not a known-plaintext attack! In a known-plaintext attack, one has known-plaintext pairs and tries to determine the key. CTR mode is secure against KPA attacks and actually, that is a lesser attack than Ind-CPA.

Improve this answer
$\endgroup$
7
  • $\begingroup$ Ah... and what kind of attack would be the bit-flipping which modifies a known plaintext by flipping bits in the ciphertext? Or isn't this an attack, because it is trivial? $\endgroup$
    – MichaelW
    Commented Feb 7, 2022 at 17:55
  • 1
    $\begingroup$ In general terms, it is an active attack that modifies the ciphertext. In specific, it is a bit-flipping attack even CBC mode has bit flipping attack $\endgroup$
    – kelalaka
    Commented Feb 7, 2022 at 17:59
  • $\begingroup$ But anyway, in practical terms I can state, that GCM without GMAC is not secure when the plain text can be guessed? Right? $\endgroup$
    – MichaelW
    Commented Feb 7, 2022 at 18:02
  • 1
    $\begingroup$ Guessing the message makes the attack easier in the case if the IV-reuse under the same key. This will reveal the other message not the key. $\endgroup$
    – kelalaka
    Commented Feb 7, 2022 at 18:19
  • 1
    $\begingroup$ The games we played in the Ind-CPA, Ind-CCAx we talk about a single bit with multiple tries of the adversary. We expect that the advantage of the adversary is $1/2+\epsilon$ where $\epsilon$ is a negligible amount. So the adversary has only a negligible chance of success. $\endgroup$
    – kelalaka
    Commented Feb 7, 2022 at 19:01

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.